Finding the sides of a $30^\circ$$60^\circ$$90^\circ$ triangle with hypotenuse $7\sqrt{3}$ closed Ask Question Finding the area of a $$ triangle with the length of the hypotenuse included without using trigonometric functions Hot Network Questions👉 Learn about the special right triangles A special right triangle is a right triangle having angles of 30, 60, 90, or 45, 45, 90 Knowledge of the ratio oAnswer (1 of 3) How do I find the missing sides in special right triangles using the 30–60–90 rule?
The Easy Guide To The 30 60 90 Triangle
Find sides of 30 60 90 triangle
Find sides of 30 60 90 triangle- The triangle is a special right triangle, and knowing it can save you a lot of time on standardized tests like the SAT and ACT Because its angles and side ratios are consistent, test makers love to incorporate this triangle into problems, especially on the nocalculator portion ofWatch more videos on http//wwwbrightstormcom/math/geometrySUBSCRIBE FOR All OUR VIDEOS!https//wwwyoutubecom/subscription_center?add_user=brightstorm2VI



How To Work With 30 60 90 Degree Triangles Education Is Around
Have no fear, in this excellent video, Davitily from Math Problem Generator explains the process step by step using easy to follow examples The video covers common examples and tricky snags that you are likely to encounter on your next math class exam All degree triangles have sides with the same basic ratio Two of the most common right triangles are and degree triangles If you look at the 30–60–90degree triangle in radians, it translates to the following In any triangle, you see the following The shortest leg is across from the 30degree angle The sides of a right triangle lie in the ratio 1√32 The side lengths and angle measurements of a right triangle Credit Public Domain We can see why these relations should hold by plugging in the above values into the Pythagorean theorem a2 b2 = c2 a2 ( a √3) 2 = (2 a) 2 a2 3 a2 = 4 a2
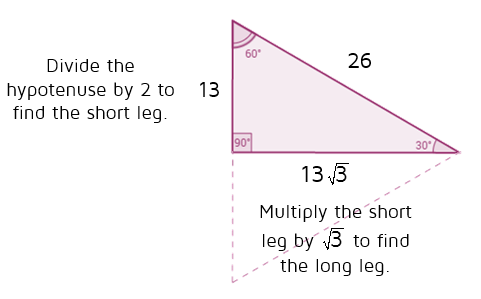
Question Find the remaining sides of a 30° – 60° – 90° triangle if the longest side is 9 The side opposite 60° is and the shortest side isYou can put this solution on YOUR website!Multiply this answer by the square root of 3 to find the long leg Type 3 You know the long leg (the side across from the 60degree angle) Divide this side by the square root of 3 to find the short side Double that figure to find the hypotenuse Finding the other sides of a triangle when you know the hypotenuse
Answer to Find the remaining side of a 30 degrees 60 degrees 90 degrees triangle if the longest side is 9 By signing up, you'll get thousands30 60 90 and 45 45 90 Triangle Calculator I N S T R U C T I O N S Start by entering the length of a triangle side Then click on which type of side it is The 5 choices you have are As soon as you click that box, the output boxes will automatically get filled in by the calculator Clicking "RESET" clears all of the boxesTrigonometry (7th Edition) Edit edition Solutions for Chapter 11 Problem 54P Find the remaining sides of a 30°−60°−90° triangle ifthe side opposite




A Quick Guide To The 30 60 90 Degree Triangle Dummies




A 30 60 90 Triangle Is Shown Below Find The Length Of The Side Labeled Y Brainly Com
👉 Learn about the special right triangles A special right triangle is a right triangle having angles of 30, 60, 90, or 45, 45, 90 Knowledge of the ratio oSolve problems involving right triangles Find the length of the hypotenuse of a right triangle if the lengths of the other two sides are 4 inches and 4√3 inches Step 3 Calculate the third side Answer The length of the hypotenuse is 8 inches You can also recognize a triangle by the angles A triangle is a special right triangle (a right triangle being any triangle that contains a 90 degree angle) that always has degree angles of 30 degrees, 60 degrees, and 90 degrees Because it is a special triangle, it also has side length values which are always in a consistent relationship with one another




Relationships Of Sides In 30 60 90 Right Triangles Ck 12 Foundation




30 60 90 Triangle Theorem Ratio Formula Video
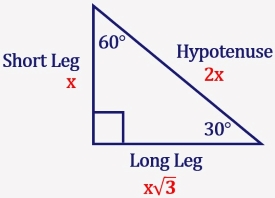
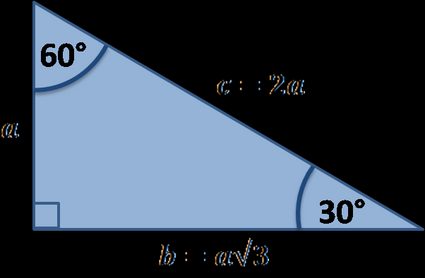
30°60°90° triangle is actually the equilateral triangle cut along the altitude The relationship between sides can be established by choosing hypotenuse as 2a The short leg (a) is opposite to 30° angle and it is half the length of the hypotenus Does your geometry homework have you stumped about finding the sides of a right triangle?Answer (1 of 3) If the length of the hypotenuse is given by r, let a = 30 degrees for now x = r*cos a y = r*sin a Then b = 60 degrees, the side between a = 30 degrees and the right angle will be x and the side between b = 60 degrees and the right angle will be y x = r*cos 30 degrees = SQRT(



1



5 5 Special Triangles
Example of 30 – 60 90 rule Example 1 Find the missing side of the given triangle As it is a right triangle in which the hypotenuse is the double of one of the sides of the triangle Thus, it is called a triangle where smaller angle will be 30 The longer side is always opposite to 60° and the missing side measures 3√3 units inAnswer to Find the remaining sides of a 30 60 90 triangle if the longest side is 9 By signing up, you'll get thousands of stepbystepAnswer (1 of 3) If we take a triangle ABC, right angled at A And assume < B = 60°, < C = 30° Then, sin 60° = opposite side / hypotenuse => √3/2 = AC/BC = √3x / 2x & By pythagoras law, BC² = AB² AC² => BC² = x² 3x² = 4x² => BC = 2x Hence, ratio of the lengths of ABACBC = 1 √3 2
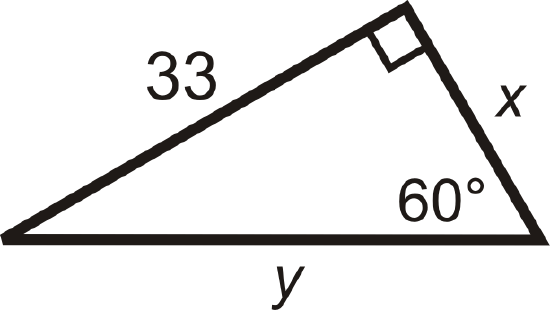



4 43 30 60 90 Right Triangles K12 Libretexts
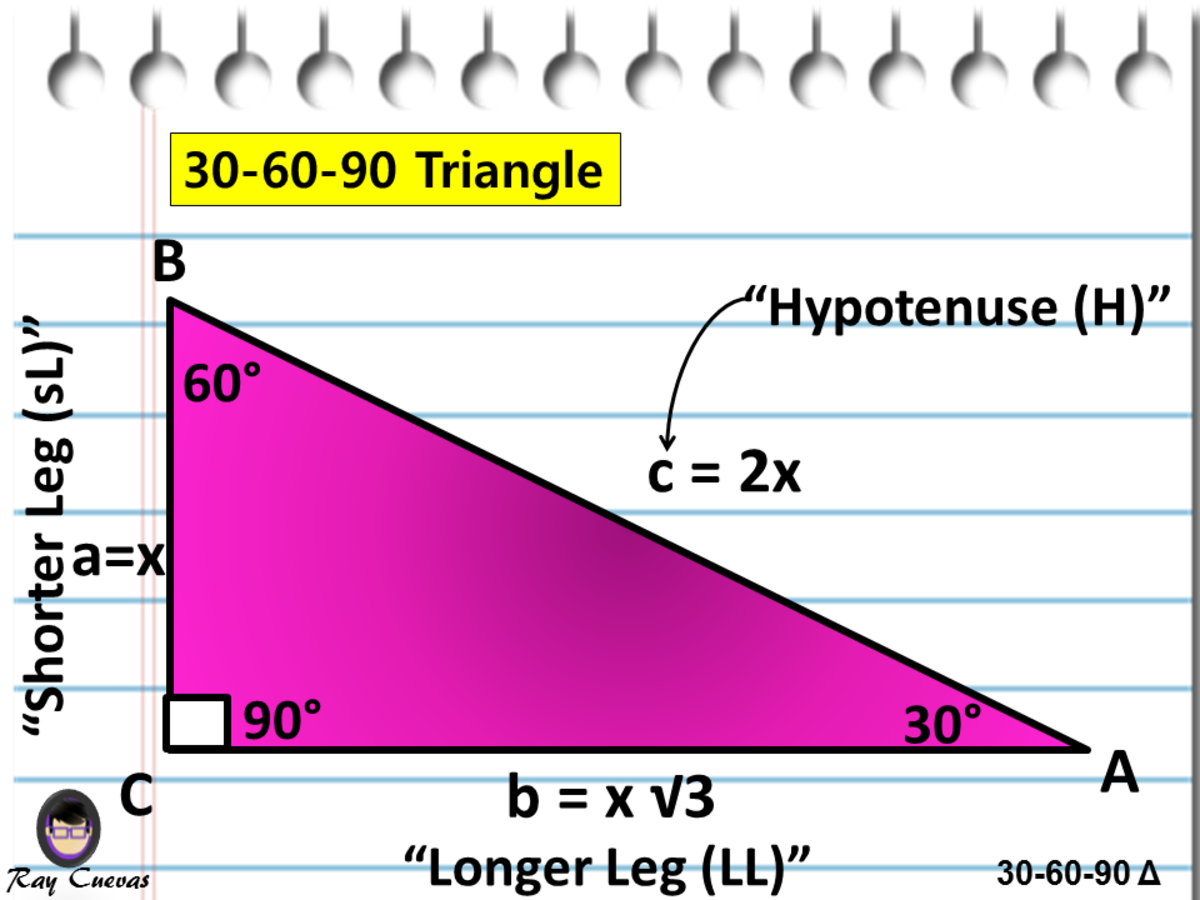



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation
It turns out that in a triangle, you can find the measure of any of the three sides, simply by knowing the measure of at least one side in the triangle The hypotenuse is equal to twice the length of the shorter leg, which is the side across from the 30 degree angleWe know from Plane (Euclidean) Geometry that in a right triangle, the length of the side opposite the 30 degree angle is equal to onehalf the length of the hypotenuse Also, from righttriangle trigonometry, we know that sin 30º = (lengtTriangle in trigonometry In the study of trigonometry, the triangle is considered a special triangleKnowing the ratio of the sides of a triangle allows us to find the exact values of the three trigonometric functions sine, cosine, and tangent for the angles 30° and 60° For example, sin(30°), read as the sine of 30 degrees, is the ratio of the side




Identifying The 30 60 90 Degree Triangle Dummies




30 60 90 Right Triangle Side Ratios Expii
12sqrt (3) is the ratio for the sides opposite degree angles The hypotenuse is 2*14=28 cm Therefore the length of every side from the standard ratio 12sqrt (3) is multiplied by 14 The three sides are 4, 4sqrt(3), and 8 The ratio of the sides in a triangle is xxsqrt(3)2x That means if the side opposite 30^circ is x, then the side opposite 60^circ is xsqrt(3) and the side opposite 90^circ is 2x Since the longest side is the hypotenuse, which is opposite 90^circ, we know that 2x = 8\rightarrow x = 4Find the remaining sides of a 30°–60°90° triangle if 39 The shortest side is 1 40 "The shortest side is 3 41 The longest side is 8 42 The longest side is 5 43 The longest side is 44 'The longest side is 24 45 The medium side is 3V3 46 "The medium side is 2V3 47 The medium side is 6 48 The medium side is 4




How To Find The Sides Of A 30 60 90 Right Triangle Math Wonderhowto




5 30 60 90 Triangles Geometry15a
Find the remaining sides of a 30°60°90° triangle if the longest side is 9 Start your trial now!A triangle is a right triangle where the three interior angles measure 30 °, 60 °, and 90 ° Right triangles with interior angles are known as special right triangles Special triangles in geometry because of the powerful relationships thatIt doesn't matter which




30 60 90 Special Right Triangle Calculator Inch Calculator




30 60 90 Triangle Formulas Rules And Sides Science Trends
Find out what are the sides, hypotenuse, area and perimeter of your shape and learn about 45 45 90 triangle formula, ratio and rules If you want to know more about another popular right triangles, check out this 30 60 90 triangle tool and the calculator for special right triangles This is a triangle with one side length given Let's find the length of the other two sides, a and b Since the side you are given, 8, isQuestion How do you find the perimeter of a triangle with only the Hypotenuse of 28cm?




30 60 90 And 45 45 90 Triangles Youtube




Special Right Triangles 30 60 90 And 45 45 90 Triangles Youtube
Without knowing our special triangle rules, we would have to use trigonometry and a calculator to find the solution to this problem, since we only have one side measurement of a triangle But because we know that this is a special triangle, we can find the answer in just secondsPractice Using the Triangle to Find Side Lengths with practice problems and explanations Get instant feedback, extra help and stepby A $$ is one of the must basic triangles known in geometry and you are expected to understand and grasp it very easily In an equilateral triangle, angles are equal As they add to $180$ then angles are are all $\frac {180}{3} = 60$ And as the sides are equal all sides are equal (see image) So that is a $$ triangle



The Easy Guide To The 30 60 90 Triangle




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com
Find the area of a triangle whose sides are 26 cm, 28 cm and 30 cm If two acute angles of a right triangle are equal,then each acute is equal to 30^(0) (b) 45^(@) (c) In a rightangled triangle ABC, angleB=90^(@), angleA=30^(@) and AC = cm Determine the lengths oWEBSITE http//wwwteachertubecom If you are given one side of a 45 45 90 triangle or one side of a 30 60 90 triangle you have enough information to solveThe right triangle is a special case triangle, with angles measuring 30, 60, and 90 degrees This free geometry lesson introduces the subject and provides examples for calculating the lengths of sides of a triangle



Solution In A Triangle Abc Angle A 30 Angle B 90 And Ab Is 12 Cm Find The Length Of
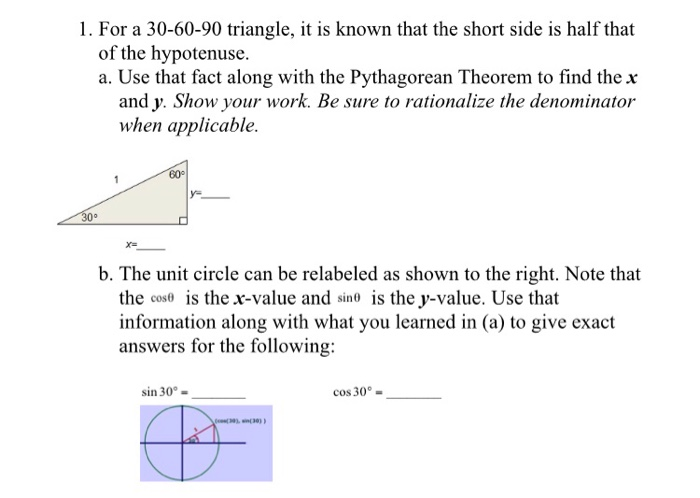



Solved 1 For A 30 60 90 Triangle It Is Known That The Chegg Com
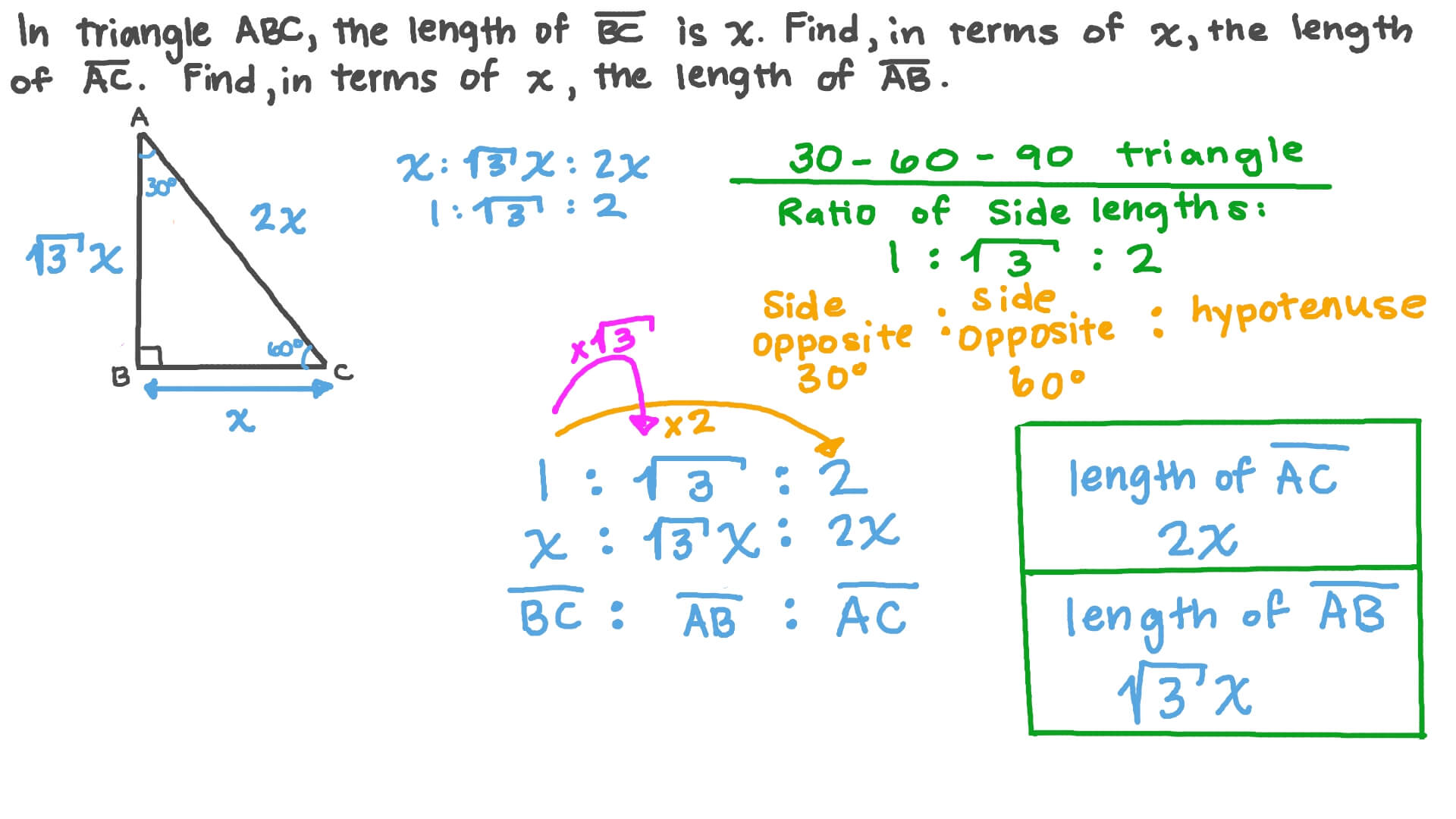
This rule only works for right triangles whose other internal angles are 30° and 60° respectively Further, for the rule to work, you need to know the length of one side;A triangle is a special right triangle with some very special characteristics If you have a degree triangle, you can find a missing side length without using theA triangle is a special triangle since the length of its sides is always in a consistent relationship with one another In the belowgiven triangle ABC, ∠ C = 30°, ∠ A = 60°, and ∠ B = 90° We can understand the relationship between each
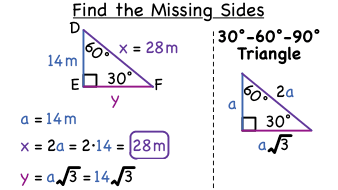



How Do You Find Missing Sides In A 30a 60a 90a Triangle Virtual Nerd




30 60 90 Formula Learn Formula For Calculating The 30 60 90 Measures
I use this activity to have my students discover the relationships between the sides on and triangles This activity can be modified by having the side lengths written on the worksheets Depending on time, sometimes I have the measurements written in and other times I have my students review measuring (cm)Triangles are classified as "special right triangles" They are special because of special relationships among the triangle legs that allow one to easily arrive at the length of the sides with exact answers instead of decimal approximations when using trig functionsAbout Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us Creators



Find The Side Lengths Of A Special Right Triangle 30 60 90 Mathondemand Com



A 30 60 90 Triangle
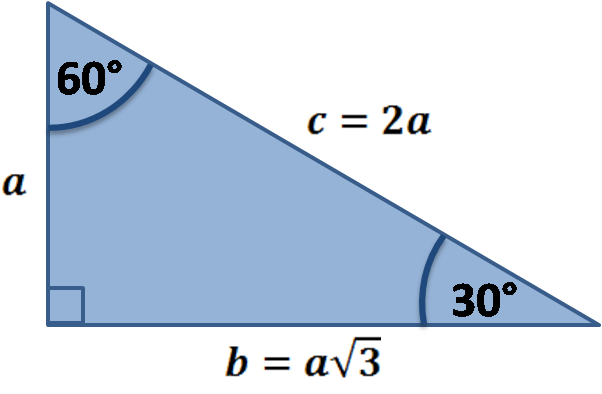
Find the remaining sides of a 30° – 60° – 90° triangle if the longest side is 9 The side opposite 60° is and the shortest side is ;A triangle is a special right triangle with some very special characteristics If you have a degree triangle, you can find a missing side length without using the 30 60 90 triangle sides If we know the shorter leg length a, we can find out that b = a√3 c = 2a If the longer leg length b is the one parameter given, then a = b√3/3 c = 2b√3/3 For hypotenuse c known, the legs formulas look as follows a = c/2



The Easy Guide To The 30 60 90 Triangle



30 60 90 Triangles P4 Kate S Math Lessons
First week only $499!THE 30°60°90° TRIANGLE THERE ARE TWO special triangles in trigonometry One is the 30°60°90° triangle The other is the isosceles right triangle They are special because, with simple geometry, we can know the ratios of their sides Theorem In a 30°60°90° triangle the sides are in the ratio 1 2 We will prove that below




Lesson 6 3 6 4 Objective To Find The Two Missing Lengths Of A 30 60 90 Triangle To Classify Four Sided Polygons In A 30 60 90 Triangle The Hypotenuse Ppt Download



1



30 60 90 Triangle Rules



Question Video The Side Lengths Of 30 60 90 Triangles Nagwa



How To Find The Legs Of A 30 60 90 Triangle Quora



3




Special Right Triangles Ppt Video Online Download



30 60 90 Triangle Calculator Formula Rules



Solve A 30 60 90 Triangle With Gradea




How To Find The Sides Of A 30 60 90 Right Triangle Math Wonderhowto




30 60 90 Right Triangles Solutions Examples Videos
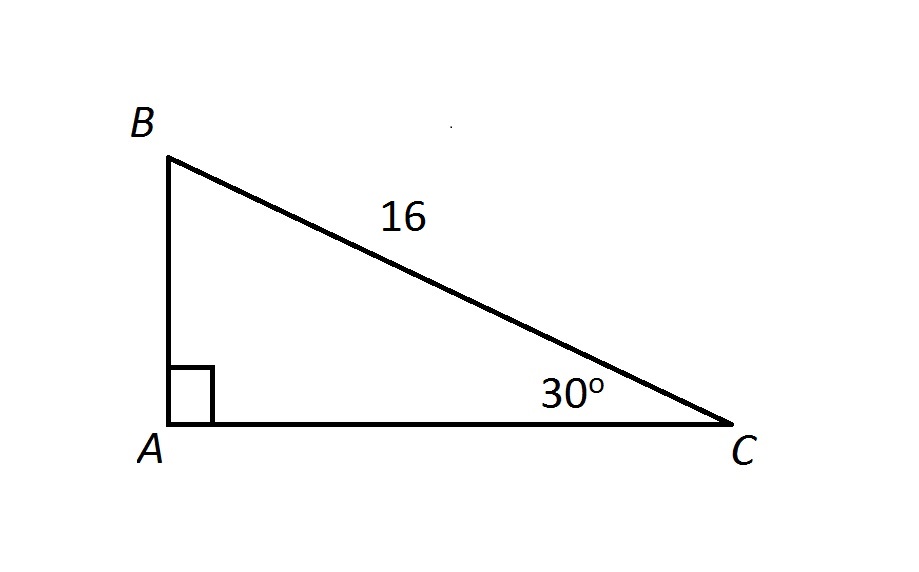



Special Triangles Hiset Math




How How Do I Find The Measure Of The Sides Of An Equilateral Triangle Inscribed In A 30 60 90 Triangle Mathematics Stack Exchange
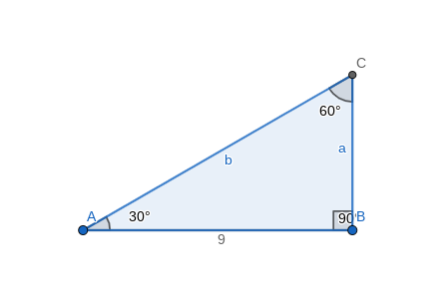



Activity 22 Construct A 30 60 90 Triangle Geogebra
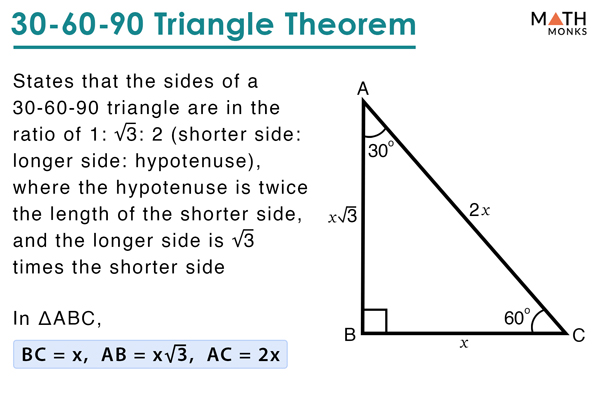



30 60 90 Triangle Definition Formulas Examples
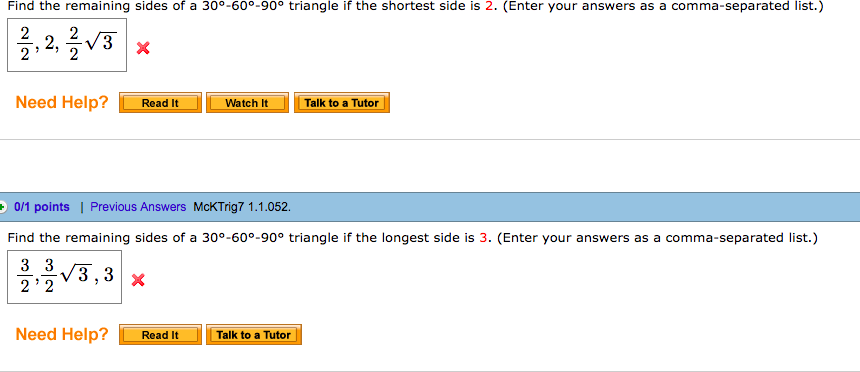



Solved Find The Remaining Sides Of A 30 60 90 Triangle If Chegg Com



How To Work With 30 60 90 Degree Triangles Education Is Around




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation
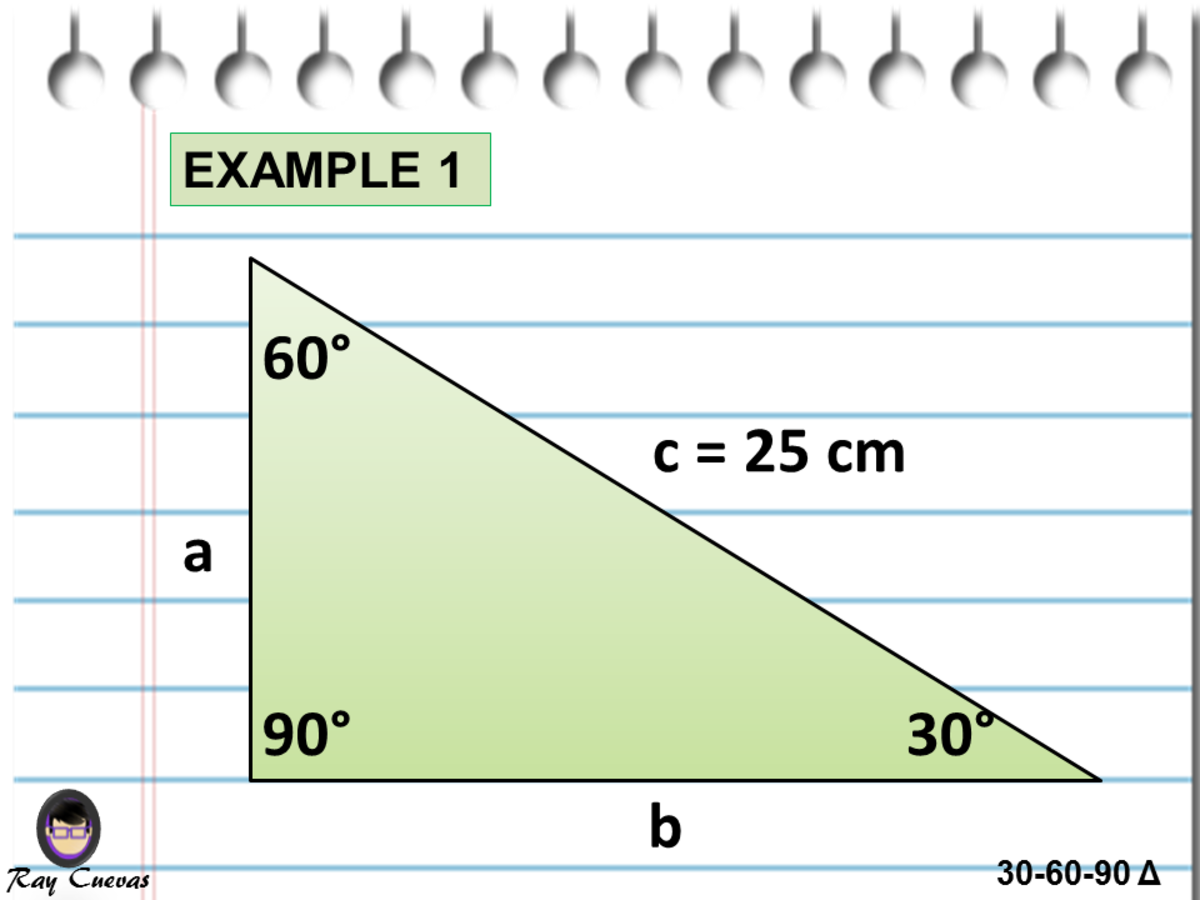



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com




30 60 90 Triangle Theorem Ratio Formula Video




How To Work With 30 60 90 Degree Triangles Education Is Around



Unit Four The Crraszy Minds
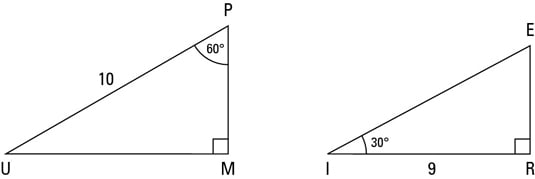



Identifying The 30 60 90 Degree Triangle Dummies




30 60 90 Triangles Spock S Guide To Math 4




30 60 90 Right Triangles Free Math Help




30 60 90 Triangle Calculator Formula Rules




30 60 90 Triangle Definition Theorem Formula Examples




30 60 90 Triangle Formulas Rules And Sides Science Trends




30 60 90 Triangle Theorem Ratio Formula Video




30 60 90 Triangle Explanation Examples




30 60 90 Triangle Example Problem Video Khan Academy




He Length Of The Hypotenuse Of A 30 60 90 Triangle Is 13 Meters Find The Length Of The Side Brainly Com




30 60 90 Triangle Explanation Examples




30 60 90 Triangle Calculator Formula Rules




How To Use The Special Right Triangle 30 60 90 Studypug




Relationships Of Sides In 30 60 90 Right Triangles Ck 12 Foundation
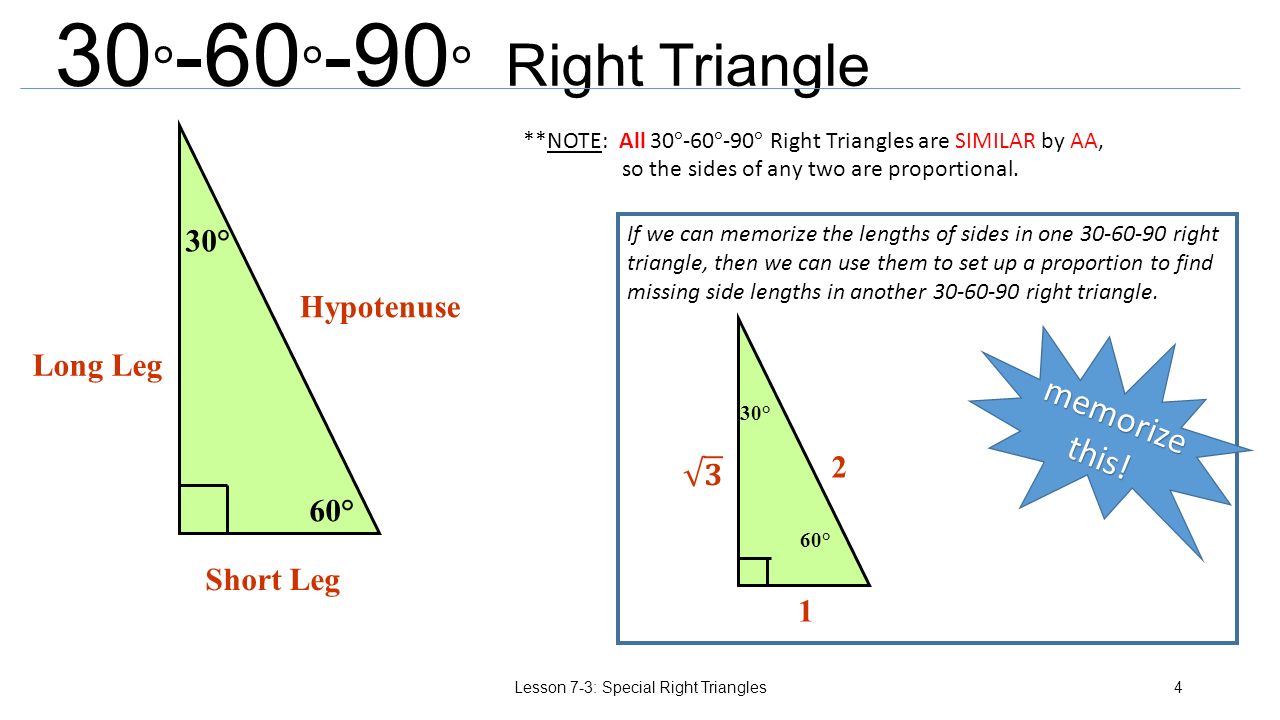



Special Right Triangles Lesson 7 3 Special Right Triangles1 Ppt Download
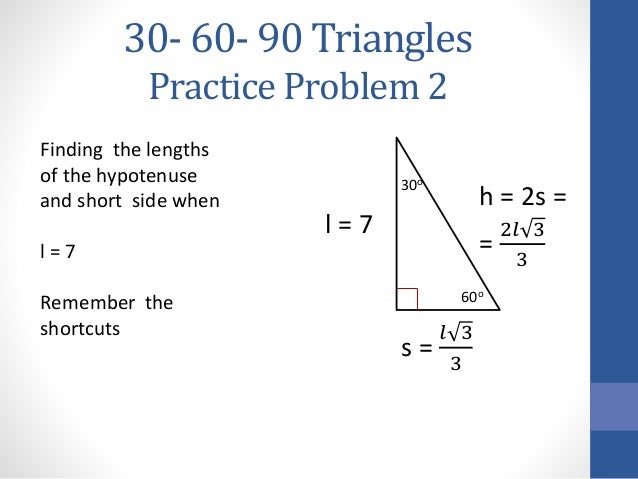



30 60 90 Triangles




Special Right Triangles Video Lessons Examples And Solutions




30 60 90 Triangles Geometry Lessons High School Math Lessons Math Lessons



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation



30 60 90 Special Triangles Geometry Mathsux 2




Relationships Of Sides In 30 60 90 Right Triangles Ck 12 Foundation
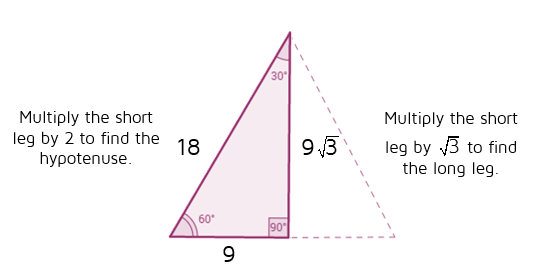



30 60 90 Triangles P4 Kate S Math Lessons



The Easy Guide To The 30 60 90 Triangle



30 60 90 Triangle Calculator Online Discount Shop For Electronics Apparel Toys Books Games Computers Shoes Jewelry Watches Baby Products Sports Outdoors Office Products Bed Bath Furniture Tools Hardware




Special Right Triangle Wikipedia




30 60 90 Triangle Explanation Examples
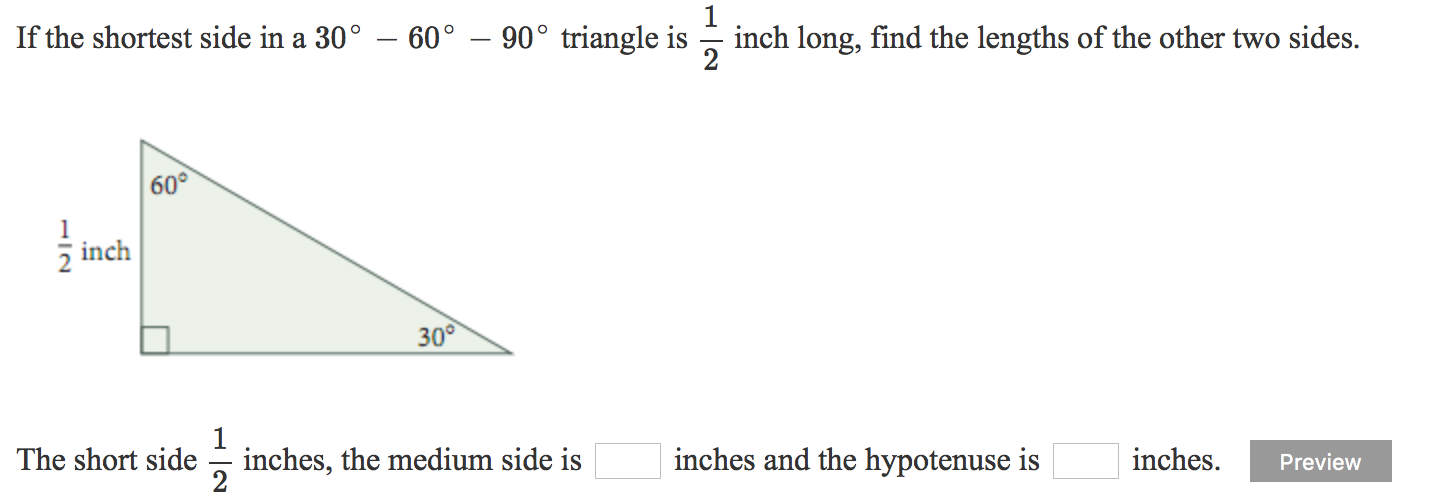



Solved If The Shortest Side In A 30 60 90 Triangle Is Z Chegg Com




Instructions Use The Ratio Of A 30 60 90 Triangle To Solve For The Variables Leave Your Answers As Brainly Com




What Is A 30 60 90 Degree Triangle Virtual Nerd
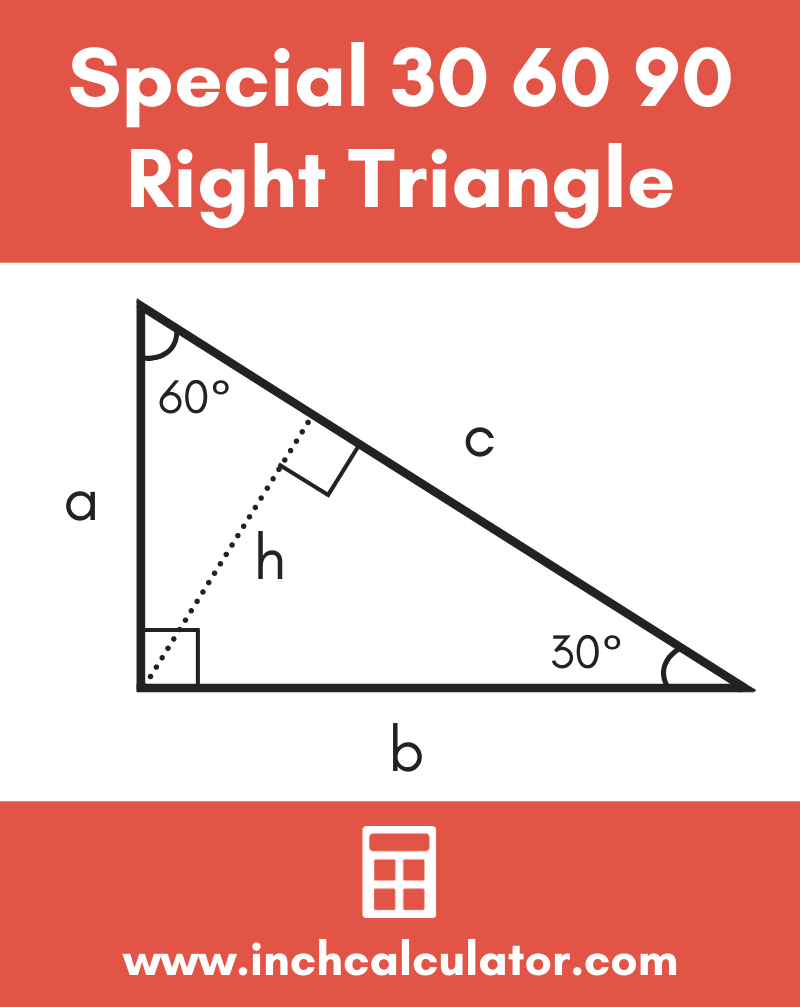



30 60 90 Special Right Triangle Calculator Inch Calculator
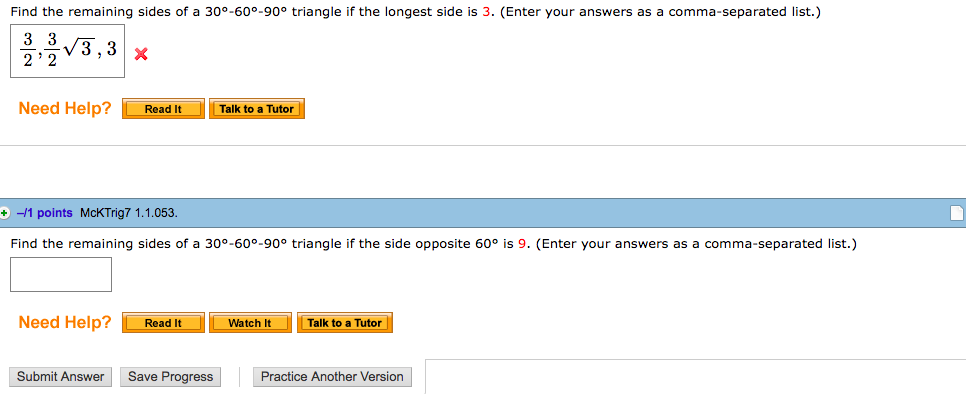



Solved Find The Remaining Sides Of A 30 60 90 Triangle If Chegg Com
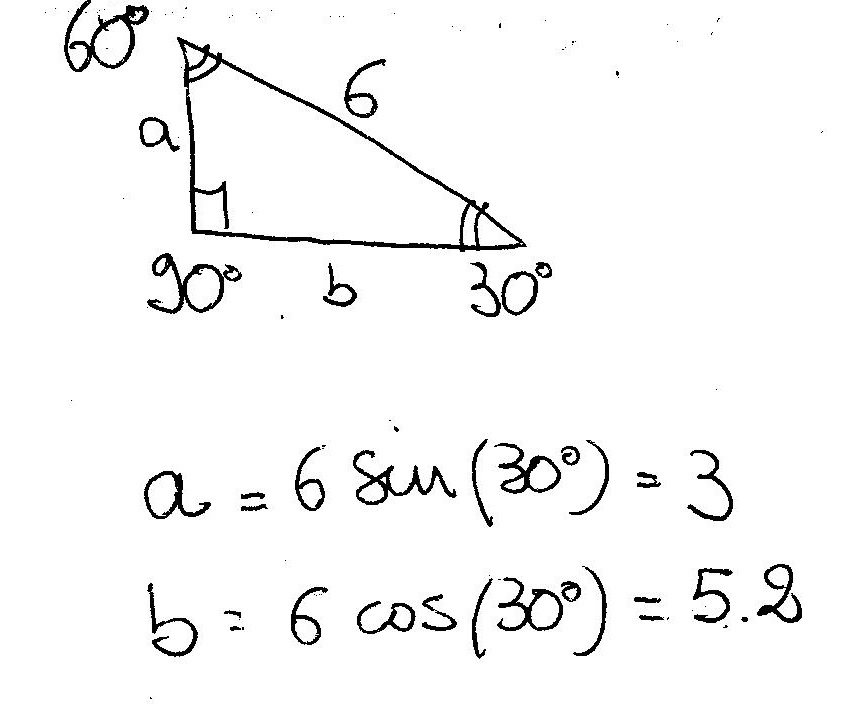



How Do You Find The Remaining Sides Of 30 Circ 60 Circ 90 Circ Triangle If The Longest Side Is 6 Socratic




The 30 60 90 Triangle Topics In Trigonometry




30 60 90 Special Triangles Geometry Mathsux 2




Ppt Bell Ringer Powerpoint Presentation Free Download Id
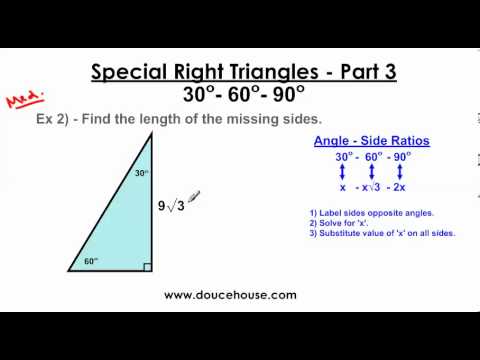



Special Right Triangles Part 3 30 60 90 Youtube



5 5 Special Triangles




30 60 90 Right Triangles Read Geometry Ck 12 Foundation
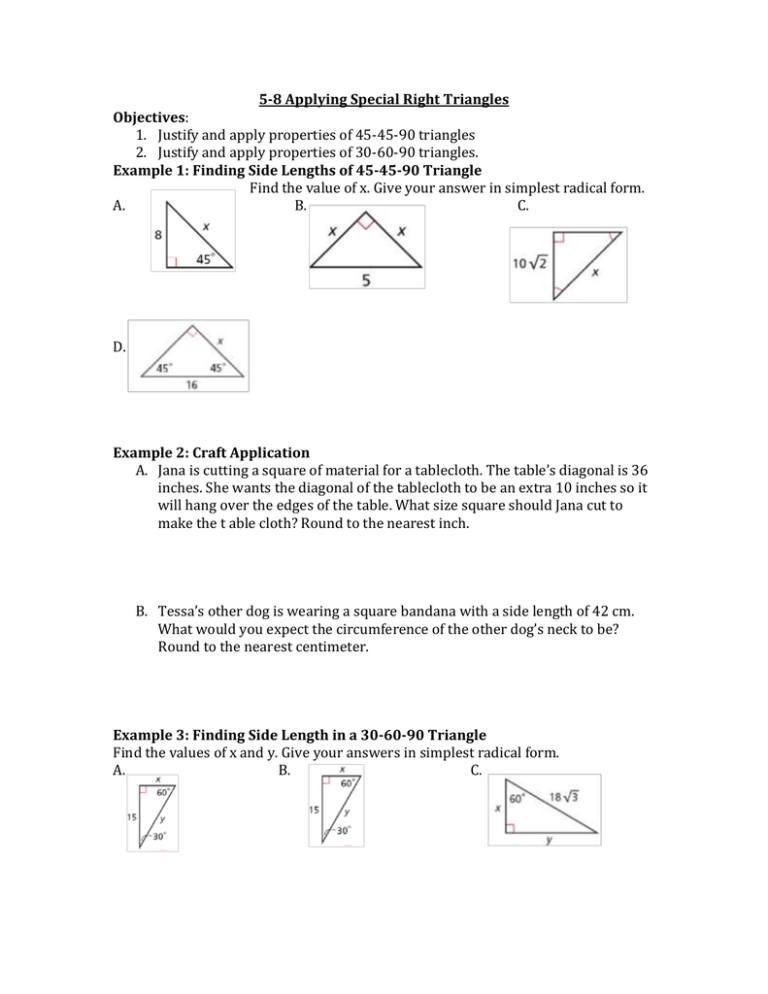



5 8 Special Right Triangles
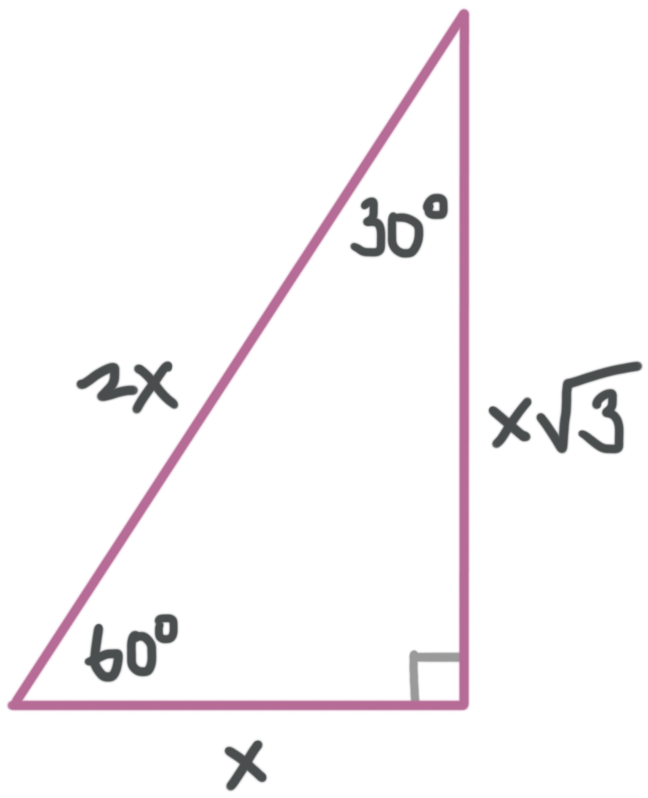



How To Solve 30 60 90 Triangles Krista King Math Online Math Tutor




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com




30 60 90 Triangle Theorem Ratio Formula Video




The Complete Guide To The 30 60 90 Triangle




View Question Practice Problems 1 In A 30 60 90 Triangle What Is The Length Of The Hypotenuse When The Shorter Leg Is 8m




30 60 90 Triangle Theorem Ratio Formula Video




A Quick Guide To The 30 60 90 Degree Triangle Dummies



The Easy Guide To The 30 60 90 Triangle



1
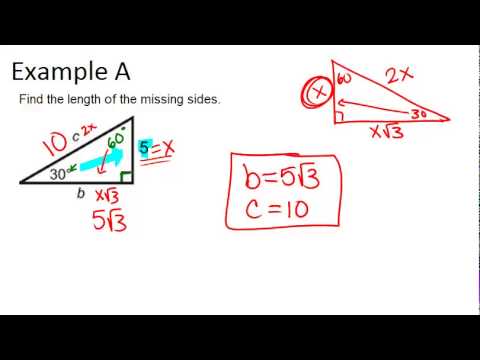



30 60 90 Right Triangles Examples Geometry Concepts Youtube




30 60 90 Triangle Theorem Ratio Formula Video




A Triangle With Angle Measures 30 60 90 Has A Perimeter Of 18 6 3 Pwn Test Prep




Finding The Missing Side Of 30 60 90 Triangle Youtube



30 60 90 Degree Triangle Shefalitayal




30 60 90 Triangle Definition Theorem Formula Examples


